Quantitative Phase Imaging & Optical Diffraction Tomography
Quantitative phase imaging

Quantitative phase imaging (QPI) is a marker-free technique for quantifying the optical thickness of cells. We are interested in optical properties that can be derived from the optical thickness, including refractive index (RI) or mass density of cells. QPI is commonly performed using digital holographic microscopy (DHM) [2]. In addition, we employ commercial QPI hardware (Phasics, S.A., France) that is based on quadriwave lateral-shearing interferometry [3]. We apply these techniques, for example, to find average RI values for single cells or cell populations and we are employing QPI to study various biological questions [4, 5].
Optical diffraction tomography
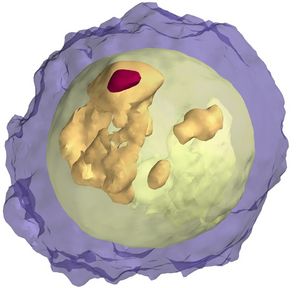
Optical diffraction tomography (ODT) is a 3D imaging technique that is used to obtain the quantitative RI distribution within cells. ODT employs QPI techniques to record 2D phase images of a cell from multiple angles which are then used for the tomographic 3D reconstruction. The tomographic approach is crucial for 3D RI imaging, because it allows to deduce the RI from the optical thickness that is measured with QPI [6, 7].
In practice, we employ optical and microfluidic devices (see Optical Trapping) to rotate single cells while acquiring QPI and fluorescence data. Our approach to ODT allows to image single cells in suspension, but requires elaborate pre-processing steps. Thus, we are constantly developing state of the art image registration and tomographic reconstruction algorithms [8, 9]. If you are interested in our QPI and ODT techniques, please check out the software section.
[1] P. Müller, “Optical Diffraction Tomography for Single Cells,” PhD Thesis, 2016.
[2] M. Schürmann, J. Scholze, P. Müller, C. J. Chan, A. E. Ekpenyong, K. J. Chalut, and J. Guck, “Refractive index measurements of single, spherical cells using digital holographic microscopy,” in Biophysical methods in cell biology, E. K. Paluch, Ed., Academic Press, 2015, vol. 125, p. 143–159.
[3] S. Mousset, C. Rouyer, G. Marre, N. Blanchot, S. Montant, and B. Wattellier, “Piston measurement by quadriwave lateral shearing interferometry,” Optics Letters, vol. 31, iss. 17, p. 2634–2636, 2006.
[4] M. Schürmann, J. Scholze, P. Müller, J. Guck, and C. J. Chan, “Cell nuclei have lower refractive index and mass density than cytoplasm,” Journal of Biophotonics, p. pulished online prior to press, 2016.
[5] P. Müller, M. Schürmann, S. Girardo, G. Cojoc, and J. Guck, “Accurate evaluation of size and refractive index for spherical objects in quantitative phase imaging,” Optics express, vol. 26, iss. 8, p. 10729–10743, 2018.
[6] P. Müller, M. Schürmann, and J. Guck, “The Theory of Diffraction Tomography,” arXiv 1507.00466 [q-bio.QM], 2015.
[7] P. Müller, M. Schürmann, and J. Guck, “ODTbrain: a Python library for full-view, dense diffraction tomography,” BMC Bioinformatics, vol. 16, iss. 1, p. 1–9, 2015.
[8] P. Müller, M. Schürmann, C. J. Chan, and J. Guck, “Single-cell diffraction tomography with optofluidic rotation about a tilted axis,” in Proceedings of the international society for optics and photonics, 2015, p. 95480U.
[9] M. Schürmann, G. Cojoc, S. Girardo, E. Ulbricht, J. Guck, and P. Müller, “Three-dimensional correlative single-cell imaging utilizing fluorescence and refractive index tomography,” Journal of biophotonics, vol. 11, iss. 3, p. e201700145, 2017.