Cavity Optomechanics: Brief introduction
Radiation pressure – Four hundred years ago, the astronomer Johannes Kepler wrote a book about comets. He noted that the tail of a comet always points away from the sun. His explanation: The light emanating from the sun pushes away the tail. This is probably the first known speculation about the physical phenomenon of radiation pressure. It was only Maxwell, though, who came up with a quantitative prediction for the radiation pressure force, and it took until 1901 to be detected in the laboratory (by Nichols and Hull in Dartmouth and by Lebedev in Moscow). Since then, radiation forces have been observed and exploited in various settings, for example in controlling the motion of cold atoms or dielectric particles. However, usually the light only provides some fixed forces, while there is no backaction of the mechanical motion onto the light field. This changes when we turn to the field of optomechanics, where there is a true two-way interaction.
Perhaps the most impressive precision measurement of all time is the detection of gravitational waves using kilometer-length Michelson interferometers. Using the two LIGO detectors, gravitational waves from the inspiral of two black holes have been measured in September 2015. These instruments are so sensitive that they can measure displacements of the order of the size of a nucleus. Their noise approaches the ultimate limits set by quantum mechanics. These limits arise due to the unavoidable shot noise of the laser beam. When the light power is rather low, the measured phase shift is uncertain due to the limited number of photons. As the power increases, the relative fluctuations of photon number become smaller, and the measurement gets more precise. However, there is a limit to the precision. At some point, for higher laser powers, another effect kicks in, and this is directly related to radiation pressure. The unavoidable photon shot noise fluctuations of the laser beam exert a random force on the end mirrors of the interferometer, which imprint some extra jitter motion that masks the real signal. Due to this “backaction noise”, the precision decreases again. As a consequence, there is an intermediate power at which some optimum is reached, and that is known as the “standard quantum limit” of displacement detection. The importance of radiation pressure noise for the sensitivity of LIGO-type detectors was already discussed in the 70s, and in the 80s it led researchers to consider the use of “squeezed light” (which will become part of some future LIGO version).
LIGO is an example of a cavity-optomechanical system, albeit with rather unusual dimensions. About 10 years ago it was realized that many nano- and microscale systems can also exhibit optomechanical effects, and that these can be very strong. Since then, there has been a growing worlwide community of researchers that tries to harness the radiation pressure force (and similar radiation forces) for applications such as ultrasensitive detection, quantum communication, and studies in the foundations of quantum physics. Incidentally, modern research into cavity optomechanics at the micro- and nanoscale is also feeding back into improved ideas for gravitational wave detection.
Cavity optomechanics setup – The typical system in cavity optomechanics is some optical cavity whose geometry changes due to any kind of mechanical motion. This might be the motion of an end-mirror, the motion of some polarizable object inside the cavity, or the deformation of a dielectric boundary (in a whispering gallery mode optical resonator or photonic crystal). If this description sounds very generic, then that is because indeed practically any optical cavity system will display optomechanical effects. The only question is how large they are, which depends on the details of the geometry but also on the amplitude of the mechanical motion. Having a cavity strongly enhances the effects, as compared to a simple free-space system with light bouncing off a mirror in the absence of any cavity. First of all, the incoming laser intensity is resonantly enhanced. It is multiplied by the finesse of the cavity, which can be on the order of a million. This increases the radiation force by the same amount. At the same time, tiny changes in the boundary of the cavity will induce a shift of all its optical resonance frequencies. Suppose the laser had initially been in resonance with the cavity, filling it up with light. A small shift of the resonance now will lead to a rapid decrease of light intensity, which will also decrease the force. It is in this way that light does not only exert a force, but any resulting motion will act back on the light field and modify the force in turn. There is a true interaction between light and mechanical motion, and this is at the heart of optomechanics.
One of the remarkable facts about optomechanics is the huge variety of physical devices in which it can be observed. Masses range from picogram nanodevices to kilogram-scale macroscopic mirrors, length scales from micrometer to centimeter (and beyond, if we include the km-length LIGO cavities), and the mechanical frequencies span many orders of magnitude as well, from Hz to several GHz. Crucially, the frequency of the radiation does not need to be resonant with the mechanical frequencies. This adds a lot of flexibility, allowing to employ optical radiation as well as microwave radiation. It also enables one of the potential significant applications of optomechanics in the domain of quantum information processing, namely coherent conversion of quantum signals from microwave to optical frequencies.

A brief history of optomechanics – The present research field of cavity optomechanics in micro- and nanoscale systems really started to take off only in around 2006. Nevertheless, over the preceding decades there had been a number of pioneering contributions. At the end of the 60s and during the 70s, Vladimir Braginsky was studying the measurement of small signals, with part of the motivation being gravitational wave detection. He built the first microwave cavity optomechanical system and showed that the microwave radiation can lead to some extra damping (or antidamping) of the mechanical motion of an end-mirror. In the 80s, the Walther lab at MPQ in Munich were the first to observe radiation pressure effects due to optical radiation, in a setup with a macroscopic mirror whose position showed bistability under the influence of the light. During the 90s, optomechanical experiments were primarily carried forward by the group around Antoine Heidmann at LKB in Paris, and there were some first speculations about quantum effects in such systems (groups of Knight and Tombesi). From about 2004 onward, a variety of different micro- and nanoscale systems were observed to show optomechanical effects, and the number of research groups has been growing quickly ever since that time.
Reviews – A more detailed discussion can be found in either one of our two reviews. A rather early, didactical brief overview in Marquardt and Girvin, “Optomechanics” (Physics ’09); and a comprehensive review in Aspelmeyer, Kippenberg, and Marquardt, “Cavity Optomechanics” (Rev Mod Phys ’14)
The following sections contain a review of our contributions to the field, organized by topic.
Our Work
The past years have seen an explosion of interest in the interaction of light with nanomechanical motion. Typical systems contain a laser-driven optical cavity, being coupled via radiation forces to mechanical motion. The goals of this field range from foundational questions to applications in quantum information processing and in the ultrasensitive detection of mass, force, position and acceleration. Our significant early contributions to the initial developments of this field consisted in predicting the nonlinear dynamics, pointing out the requirements for ground-state laser cooling, and predicting the so-called strong coupling regime, where photons and phonons hybridize to form new excitations.
More recently, we have gone beyond the canonical optomechanical system and studied systems where many optical and mechanical modes couple to each other, forming optomechanical arrays and circuits. There, we are studying the many-body dynamics of photons and phonons interacting with each other, possibilities for mechanical quantum state processing, classical synchronization physics, and questions related to enhancing the coupling strengths.
Classical Nonlinear Dynamics

The interaction of radiation with the mechanical motion of some object can give rise to intricate nonlinear dynamics. This holds for any standard optomechanical setup, where both the intensity of the radiation and its sensitivity to the mechanical motion are amplified by exploiting an optical cavity. Beyond a certain threshold in input laser power, the mechanical resonator may suddenly start to oscillate by itself, extracting the required energy from the radiation field. This is similar to lasing action, but here the result is a self-amplified mechanical oscillation, pumped by the optical field. The existence of a linear instability threshold itself was known already (particularly as a potential nuisance in interferometric gravitational wave detection), when we started our analysis. This led to our finding a rather surprising attractor diagram, with many possible nonlinear attractors for the long-time dynamics of the system (arXiv Feb 05, PRL 06). Nowadays, it is acknowledged that this describes the generic nonlinear behaviour of optomechanical systems. The attractor diagram has been observed in experiments by now. As pointed out in our 2006 PRL, the nonlinear attractor diagram might also be exploited in ultrasensitive ‘latching’ measurements, a proposal yet to be implemented.
We later extended this analysis in several ways. We showed how the system behaves in the deep quantum regime, and we also showed that when several such limit cycle oscillators are coupled, this may give rise to optomechanical synchronization.
Laser Cooling and Strong Coupling Regime
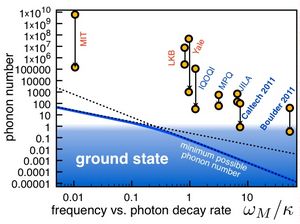
Starting in 2006, the primary goal in optomechanics, for several years, was to laser-cool the motion of the mechanical resonator. This is necessary to reach the quantum regime, since none of the mechanical resonators being used is already near the ground state at the typical bulk temperatures. In 2007, we published the complete theory of optomechanical laser-cooling, establishing the quantum limits to the phonon occupancy (arXiv Jan 07, PRL 07). This served as a theoretical foundation for all the subsequent experiments that tried to cool down to the quantum ground state of motion. This goal was finally achieved in 2011, using both optical radiation and microwaves.
In the same work, we also predicted what is now known as the strong coupling regime of optomechanics, where the photon and the phonon mode hybridize strongly, to form new excitations. This is possible if the effective (laser-enhanced) coupling rate between light and vibrations surpasses the photon decay rate. The effect is visible in the mechanical (and in the optical) spectrum, where some normal-mode splitting develops. Nowadays, this regime has been reached experimentally and is being exploited for state transfer between optical and mechanical excitations.

One of the most exciting aspects of physics is the emergence of novel complex behaviour when one combines many equivalent simple building blocks in a simple way, for example by stacking atoms to form a crystal lattice. From the theoretical perspective, this leads us into the domain of (classical or quantum) field theories, and to concepts such as phase transitions, universality, and nonequilibrium pattern formation. For this reason, engineering of periodical arrays of simple constituents is now a major goal in a number of research fields. For example, optical lattices allow to control the transport of cold atoms, arrays of superconducting cavities promise to do the same for microwave photons on a chip, and on the more microscopic scale, engineered metamaterials serve to produce novel optical properties.
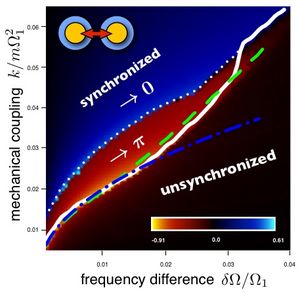
In 2009, we began investigating the dynamics of periodic arrangements of optical and vibrational modes, which we termed “optomechanical arrays”. This line of research was inspired by the newly available platform of photonic crystals (optomechanical crystals), though the concept could also be implemented in many other possible platforms, such as arrays of coupled disk resonators or arrays of microwave optomechanical systems. Our first work in this direction (arXiv Jul 10, PRL 11) introduced for the first time the concept of optomechanical arrays of this kind. Moreover, we predicted their nonlinear behaviour, especially the synchronization between optomechanical oscillators. Several years later, optomechanical arrays are now being implemented. For example, we are working with the group of Oskar Painter at Caltech, who pioneered photonic crystals in optomechanics (optomechanical crystals), to realize optomechanical arrays in this platform.
By now, optomechanical synchronization has been experimentally observed by the Tang group in Yale and by the Lipson group (Cornell/Columbia), in both cases for the simplest possible system (two coupled oscillators). The Lipson group also extended this (in 2015) to a small array of seven disk oscillators. Mathematical work by the Milburn group has extended our theoretical analysis to optical coupling between the oscillators. In general, such synchronization of oscillators could become important for metrological purposes, to suppress the effects of noise on the phase stability of the oscillations. In addition, it is very interesting from a theoretical point of view. Ultimately, such systems are described by stochastic nonlinear classical field theories on a lattice, which are of considerable interest in many areas of physics. In more recent work, we described the intricate spiral patterns that could form in the phase field that describes the oscillation phases in any 2D array of coupled limit-cycle oscillators (PRE 15).
Going from classical nonlinear dynamics to quantum nonlinear dynamics, one can study aspects of quantum many-body physics of photons and phonons. Interestingly, the full interacting Hamiltonian of an optomechanical array in the quantum regime is formally equivalent to the Holstein-Hubbard Hamiltonian in solid-state physics, except that electrons have been replaced by photons. This replacement, of course, gives rise to significant physical differences, since we are now dealing with bosons, their number is no longer conserved, and we have to face a nonequilibrium situation by necessity (the system has to be driven by a laser). The resulting model does not only show a kind of superfluid-Mott-insulator transition for the photons, but also a quantum synchronization transition. Here, synchronization of the mechanical oscillators can be disrupted by the unavoidable nonequilibrium quantum noise in the system (PRL 13). The precise nature of this phase transition is not yet completely understood, but quantum synchronization is by now becoming an active area of research.
Even in the linear regime, optomechanical arrays turn out to be surprisingly interesting. This is because the resulting bandstructure of photons and phonons can be tuned optically. Upon application of a strong pump laser field, the original nonlinear optomechanical interaction turns into a quadratic coupling whose strength and phase can be set by the laser. In this way, optomechanical arrays can (in some ways) become for photons and phonons what optical lattices are for cold atoms: a tuneable medium in which the transport can be controlled by an external laser field.
To name one example, we have shown how an optomechanical array with a honeycomb lattice could produce several of the surprising effects that have been observed for electrons in graphene, such as ultrarelativistic dynamics near the Dirac point and Klein tunneling (NJP 15). The setting would be significantly different from graphene electronics, in that we are dealing with two physically different species of (bosonic) particles, photons and phonons, which can hybridize and from new photon-phonon polaritons, with optically tuneable properties.
On the theoretical level, in our quest to understand the dynamics of optomechanical arrays we are employing direct numerical simulations of the classical nonlinear stochastic dynamics, quantum mean-field approaches (like dynamical Gutzwiller), truncated Wigner approximation methods, perturbation theory and other quantum many-body techniques. At the same time, we also care about the direct implementation in experiments, and we employ finite-element simulations to design suitable photonic crystal structures with the desired electromagnetic and acoustic properties.
Topological Transport of Light and Sound

Optomechanical arrays offer a very flexible way to control the transport of photons and phonons, most of all because their behaviour is not fixed by the geometry but can be tuned by a laser. In a series of works, we have shown how to exploit this flexibility to produce topologically protected transport of photons and phonons on a lattice.
One of the beautiful aspects of physics is the universality of certain concepts. For example, waves are ubiquitous in nature and can arise in many different forms. Even though the details of the corresponding wave equations may differ, many features carry over from one example to the next. Linear waves in periodic media will always display a bandstructure. Most aspects of bandstructures have been understood since the early days of quantum mechanics. Therefore, it is quite surprising that the concept of topological properties in bandstructures is relatively recent. It was first discovered in the context of the quantum Hall effect and then more recently became a central piece of modern physics, when a class of new materials was found which realize a slightly different version of the same concept. These are the so-called topological insulators. Physically, in all of these cases the most important aspect is that there are ‘chiral’ edge channels near the boundary of any sample made of this material. The particles (matter waves) flow along such edge channels only in one way and are robust against disorder scattering, because there is no counterpropagating edge channel. It is obvious that such a behaviour can become very helpful for many possible applications.
The optomechanical interaction between photons and phonons can often be ‘linearized’ to a very good approximation. Then it looks like a coherent conversion between photons and phonons, with a conversion amplitude that can be tuned by the strong driving laser. For our story here, it is crucial that this conversion amplitude (coupling) can also have a phase. Physically, this phase is set by the optical phase of the driving laser. That can now be exploited to produce “non-reciprocal” behaviour: A phonon or photon hopping around a circle (in a set of coupled mechanical or optical modes) can pick up an overall phase that depends on the direction of motion (clockwise vs. counter-clockwise). This phase can be identified formally with the Aharonov-Bohm phase that a charged particle would pick up when moving around a closed loop in a magnetic field. That reasoning can also be turned around. If there is such a phase, then the particle effectively feels a magnetic field, even when it is not charged. Any physical effect we usually associate with the motion of a charged particle in a magnetic field will then be present. For example, the trajectory of the particle (or the motion of a wave packet) will be curved by a Lorentz force.
During the past several years, various ideas have been put forward to produce artificial magnetic fields for photons. These ideas include particular fixed geometrical arrangements of ring resonators, magneto-optic materials, and time-dependent modulation of the dielectric properties of a photonic crystal via nearby electrodes. We were able to show that the optomechanical interaction can be used to produce the same effects but with much more flexibility. The ingredients are just a suitable photonic crystal and two laser beams with the right spatial pattern of optical phases (Optica 15). The effective photon magnetic field can then be tuned arbitrarily by variation of the driving laser intensity and phase pattern. More recently, we showed that the effective magnetic field could even become a dynamical gauge field, being determined by the mechanical oscillation phases of mechanical resonators (arXiv 15).
After the power of the concept of topological transport had been fully appreciated for electrons, researchers moved to explore its features for other systems like cold atoms, photons, or magnons. Phonons, however, were for a long time absent from this list. It is, indeed, not trivial to engineer the suitable non-reciprocal phases for phonon transport. In 2014, we were the first to come up with a proposal for engineering phonon topological transport (arXiv Sep 14, PRX 15). A short while later, other proposals were put forward and at the same time macroscopic setups using cleverly coupled pendula were produced as proof-of-principle experiments. Nevertheless, our optomechanical approach to this challenge remains the most flexible one. The optical laser phase pattern illuminating the sample determines the topological phase, and spatial variations in this pattern could be used to produce several adjacent topologically different regions (with chiral edge states in-between). Moreover, even quench physics could easily be explored in such a system, by changing the driving laser. Once realized, such optomechanical phonon Chern insulators could be exploited for studying heat transport on the nanoscale in new ways, with unidirectional edge channels connecting different heat reservoirs.
Measurement and Quantum Noise
One of the primary possible applications of optomechanical systems consists in ultrasensitive measurements, of small displacements, forces, masses, and accelerations.
It is straightforward to measure the mechanical displacement in an optomechanical system. After all, the optical cavity represents an interferometer. However, the so-called standard quantum limit for displacement detection prescribes that there is a lower limit to the unavoidable noise in such a setting. Ultimately, this arises due to Heisenberg’s uncertainty relation, because a weak continuous position measurement essentially attempts to measure the full trajectory of a quantum particle, which is of course not possible with arbitrary precision (see e.g. our review RevModPhys 10). This challenge can be circumvented, however, if one deliberately measures only a particular “quadrature” of the mechanical oscillation. We analyzed such a scheme in detail (NJP 08), and by now this has been shown in experiments (e.g. by the Schwab group).
Apart from the mechanical displacement, it would be even more interesting to be able to measure the quantized phonon number of a mechanical resonator. In 2008, in a joint work with the Harris group (Nature 08) we pointed out how that would become possible in a novel optomechanical system where the optical frequency couples to the square of the position (instead of just linearly). That would enable a quantum non-demolition measurement of phonon number, which would eventually allow to observe quantum jumps between discrete mechanical Fock states. It has to be pointed out, though, that this goal still remains very challenging, even with the present-day strongly improved systems. The reason is that it effectively requires the single-photon strong coupling regime, where the bare coupling is larger than the photon decay rate.

The optomechanical interaction between photons and phonons is fundamentally nonlinear, since the force acting on the mechanical resonator is proportional to the intensity of the light field (and not its amplitude). Nevertheless, the coupling constant is usually so small that one has to compensate for this by boosting the effects through a large laser drive. In that case, the interaction becomes linear and the more subtle effects are lost. Recent years have seen strong experimental efforts to increase the bare optomechanical coupling, such that it would become strong even at the level of single photons and single phonons. In our theory work, we have been investigating which effects would become possible if the coupling rate were to reach or exceed other important scales in the problem (such as the photon decay rate or the mechanical frequency).
One of the natural questions to ask in this context is how the classical nonlinear dynamics smoothly transitions into the quantum regime (the classical-quantum transition) when the coupling is so large that nonlinear effects can be seen even for small numbers of photons and phonons. We predicted that one will be able to see non-Gaussian (NJP 08 ) and even non-classical (PRL 12) quantum states of mechanical motion in this “single photon strong coupling” regime (as it came to be known).
Another, very relevant, question is how to increase the effective optomechanical coupling such that true quantum many-body effects could be seen in the experiments. We found out that one can engineer a system with two suitable optical modes so as to boost the quantum nonlinear effects (PRL 12). This would allow effective photon-photon interactions, as well as QND measurements of photon and phonon number. One can also turn the question around and ask how the first subtle effects of quantum nonlinearities would manifest themselves even if the coupling is not yet very strong. We predicted that in a two-tone spectroscopy experiment, the sharp spectral dips that are associated with “optomechanically induced transparency” could provide such a first tell-tale sign of nonlinearities on the quantum level (PRL 13). All of these effects still remain to be seen in experiments. A number of experimental groups are working hard to improve the designs of their physical setups to approach the strong single-photon coupling regime.






